Matrix Product States

Matrix Product States (mps) are an efficient approximation for a general weakly-entangled quantum state. The main idea is to approximate a general quantum state by a sum of simple product states. This sum of product states is generated by a matrix product of $d\cdot n$ matrices, whereby $n$ denotes the number of physical particles (e.g. spin,electrons) with local dimension $d$ (e.g. spinup/spindown). The following introduction to mps follow the review article “The density-matrix renormalization group in the age of matrix product states” by Ulrich Schollwöck¹⁾ and the bachelor thesis of Thomas Köhler.

In the following we want to focus on a one-dimensional spin-1/2-chain with $L$ sites and open boundaries. On each site there are two (physical) states $\sigma_i=\{\uparrow, \downarrow\}$. Any quantum state that describes the spins on this chain can be expressed by:

\begin{align} \lvert\psi\rangle = \sum_{\sigma_1, \ldots, \sigma_L} c_{\sigma_1\ldots\sigma_L} \lvert {\sigma_1, \ldots, \sigma_L} \rangle \end{align}

The coefficients are $(c_{\sigma_1\ldots\sigma_L} \in {C})$ and their number will increase exponentially $(2^L)$ with the length of the chain. Therefore we cannot represent states for long chains as we cannot represent all coefficients. In MPS every coefficients is given by a matrix product $A_{\sigma_1}\cdot\ldots \cdot A_{\sigma_L}$, whereby each matrix belongs locally to a site (and physical index) and just has a limited number of values.

\begin{align} \lvert\psi\rangle = \sum_{\sigma_1, \ldots, \sigma_L} A^{\sigma_1}\cdot\ldots \cdot A^{\sigma_L} \lvert {\sigma_1, \ldots, \sigma_L} \rangle \end{align}

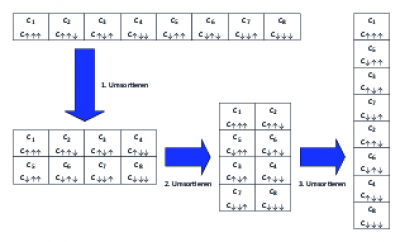
To get an MPS from a known quantum state with coefficients $c_{\sigma_1\ldots\sigma_L}$ we have to shuffle the $2^L$ coefficients into a matrix $c^\prime$ of dimension $2 \times 2^{L-1}$.

\begin{align} c_{\sigma_1\ldots\sigma_L} = c_{\sigma_1, (\sigma_2\ldots\sigma_L)}^\prime \end{align}

Now we will employ a single-value-decomposition²⁾ on the matrix $c^\prime$:

\begin{align} c_{\sigma_1, (\sigma_2\ldots\sigma_L)}^\prime = \sum_{a_1}^{r_1} U_{\sigma_1, a_1} S_{a_1, a_1} (V^\dagger)_{a_1, (\sigma_2\ldots\sigma_L)} \end{align}

Next we generate from $S$ and $V^\dagger$ a new $c_{a_1\sigma_2\ldots\sigma_L}^\prime$, that will be reshuffled into a matrix $c_{(a_1\sigma_2),(\sigma_3\ldots\sigma_L)}^{\prime\prime}$. Furthermore the matrix $U$ (with $U^\dagger U =1$) will be reshuffled into two $A^{\sigma_1}$-matrices, with coefficients: $A^{\sigma_1}_{1,a_1}=U_{\sigma_1,a_1}$. One obtains:

\begin{align} c_{\sigma_1,\ldots\, \sigma_L}=\sum_{a_1}A_{1,a_1}^{\sigma_1}c_{(a_1\sigma_2),(\sigma_3\ldots\sigma_L)}^{\prime\prime} \end{align}

One continues with an svd on $c_{(a_1\sigma_2),(\sigma_3\ldots\sigma_L)}^{\prime\prime}$. The new $S$ and $V^\dagger$ will again be combined and reshuffled into two $A$-matrices. The new coefficients are now: $A^{\sigma_2}_{a_1,a_2} = U_{(a_1\sigma_2), a_2}$. This procedure is repeated until site $L-1$ is reached. One finally obtains:

\begin{align} c_{\sigma_1,\ldots\, \sigma_L} &=\sum_{a_1}\cdots\sum_{a_{l-1}}A_{1,a_1}^{\sigma_1}\cdots A_{a_{l-2},a_{l-1}}^{\sigma_{l-1}}\underbrace{c_{(a_{l-1}\sigma_{l}), (\sigma_{l+1}\ldots\sigma_L)}^{\prime\cdots\prime}}_{M_{a_{L-1},a_L}^{\sigma_L}}\newline &=A^{\sigma_1}\cdots A^{\sigma_{L-1}}M^{\sigma_L} \end{align}

The $A$-matrices fullfill the condition for left-normalization $\sum_{\sigma_l}A^{\sigma_l\dagger}A^{\sigma_l}= 1$. One can also construct a MPS with right-normalized matrices by starting from the left and reshuffling the $V^\dagger$ into $B$-matrices, always continuing with $U$ and $S$. The $B$-matrices will the fullfill the condition for right-normalization: $\sum_{\sigma_l}B^{\sigma_l}B^{\sigma_l\dagger}= 1$. One can also generate a mixed-normalized state by starting from both sides.

The scalar product for two MPS is given by:

\begin{align} \langle \phi \lvert \psi \rangle = \sum_{\boldsymbol{\sigma\sigma^\prime}}\langle \boldsymbol{\sigma^\prime} \lvert \tilde{M}^{\sigma_1^\prime *}\cdots\tilde{M}^{\sigma_L^\prime *}M^{\sigma_1}\cdots M^{\sigma_L}\lvert \boldsymbol{\sigma} \rangle \end{align}

This can be simplified to:

\begin{align} \langle \phi \lvert \psi \rangle = \sum_{\boldsymbol{\sigma}}\tilde{M}^{\sigma_1*}\cdots\tilde{M}^{\sigma_L *}M^{\sigma_1}\cdots M^{\sigma_L} \end{align}

The computation of this expression is computationally very inefficient as one has to calculate $2^L \cdot (2L-1)$ matrix-multiplications. That means that the complexity will increase exponentially with chain length. But we can reformulate the expression, so that we just need $2L$ additions and $4L-2$ matrix-multiplications, which just scales polynomially.

\begin{align} \langle \phi \lvert \psi \rangle &= \sum_{\sigma_L}\tilde{M}^{\sigma_1\dagger}\left(\cdots\left(\sum_{\sigma_2}\tilde{M}^{\sigma_2\dagger}\left(\sum_{\sigma_1}\tilde{M}^{\sigma_1\dagger}M^{\sigma_1}\right)M^{\sigma_2}\right)\cdots\right) M^{\sigma_L} \end{align}

Analogously to quantum states, we can also express the operators as Matrix-Product-operators. We simply define:

\begin{align} \hat O & = \sum_{\boldsymbol{\sigma}, \boldsymbol{\sigma^{\prime}}} c_{(\sigma_1\ldots\sigma_L)(\sigma_1^{\prime}\ldots\sigma_L^{\prime})} \lvert {\boldsymbol{\sigma}} \rangle \langle {\boldsymbol{\sigma'}} \lvert \newline & = \sum_{\boldsymbol{\sigma}, \boldsymbol{\sigma^{\prime}}} c_{(\sigma_1\sigma_1^{\prime})\ldots(\sigma_L\sigma_L^{\prime})} \lvert {\boldsymbol{\sigma}} \rangle \langle {\boldsymbol{\sigma^{\prime}}} \lvert \newline & = \sum_{\boldsymbol{\sigma}, \boldsymbol{\sigma^{\prime}}} W^{\sigma_1\sigma_1^{\prime}}\ldots W^{\sigma_L\sigma_L^{\prime}} \lvert {\boldsymbol{\sigma}} \rangle \langle {\boldsymbol{\sigma^{\prime}}} \lvert \end{align}

The $W$-matrices will do the same job as the $M$-matrices of MPS. The difference is that they have two physical indices.

The application of a MPO on a MPS is expressed by:

\begin{align} \hat O \lvert \psi \rangle & = \sum_{\boldsymbol{\sigma}, \boldsymbol{\sigma^{\prime}}, \boldsymbol{\sigma^{\prime\prime}}} (W^{\sigma_1\sigma_1^{\prime}}\ldots W^{\sigma_L\sigma_L^{\prime}})(M^{\sigma_1^{\prime\prime}}\cdots M^{\sigma_L^{\prime\prime}})\lvert {\boldsymbol{\sigma}} \rangle \underbrace{\langle {\boldsymbol{\sigma^\prime}} \lvert {\boldsymbol{\sigma^{\prime\prime}}} \rangle}_{\delta_{\sigma^{\prime}\sigma^{\prime\prime}}}\newline & = \sum_{\boldsymbol{\sigma}, \boldsymbol{\sigma^{\prime}}} (W^{\sigma_1\sigma_1^{\prime}}\ldots W^{\sigma_L\sigma_L^{\prime}})(M^{\sigma_1^{\prime}}\cdots M^{\sigma_L^{\prime}})\lvert {\boldsymbol{\sigma}} \rangle \newline & = \sum_{\boldsymbol{\sigma}} \tilde{M}^{\sigma_1}\cdots \tilde{M}^{\sigma_L} \lvert\boldsymbol{\sigma}\rangle = \lvert \tilde\psi\rangle \end{align}

The values of the $\tilde{M}$ matrices are given by:

\begin{align} \tilde{M}^{\sigma_i} = \sum_{\sigma_i^{\prime}}W^{\sigma_i\sigma_i^{\prime}}_{b_{i-1},b_i}M^{\sigma_i^{\prime}}_{a_{i-1}, a_i} \end{align}

$\lvert\tilde\psi\rangle$ is a therefore a new MPS, but with a larger dimension. So an expectation value of an operator can be calculated by

\begin{align} \langle \psi \lvert \hat O \lvert \psi \rangle = \langle \psi \lvert \tilde \psi \rangle \end{align}

Repeated application of an operator onto a state therefore increases strongly the dimension of the state.

A general Hamilton-operator such as:

\begin{align} \hat H = \sum_{i=1}^{L-1}\frac{J}{2}\hat S_i^+\hat S_{i+1}^- + \frac{J}{2}\hat S_i^-\hat S_{i+1}^+ + J^z\hat S_i^z\hat S_{i+1}^z-h_z\sum_i^L \hat S_i^z-h_x\sum_i^L \hat S_i^x \end{align}

can be constructed analytically as an MPO. This is reasoned in the special Tensor-Product-structure of the Hamiltonian:

\begin{align} \hat H = J^z\hat S_1^z\otimes\hat S_2^z\otimes\hat I_3\otimes\hat I_4\ldots + \hat I_1\otimes J^z\hat S_2^z\otimes\hat S_3^z\otimes\hat I_4\ldots+\ldots \end{align}

To construct the Hamiltonian we have to give the matrices.

\begin{align} \hat W^{\left[i\right]}=\sum_{\sigma_i, \sigma^\prime_i}W^{\sigma_i\sigma_i^\prime}\lvert\sigma_i\rangle\langle\sigma_i^{\prime}\lvert \end{align}

They are given by:

\begin{align} \hat W^{\left[i\right]} = \begin{bmatrix} \hat I & 0 & 0 & 0 & 0 \newline \hat S^+ & 0 & 0 & 0 & 0 \newline \hat S^- & 0 & 0 & 0 & 0 \newline \hat S^z & 0 & 0 & 0 & 0 \newline -h_z\hat S^z-h_x\hat S^x & (J/2)\hat S^- & (J/2)\hat S^+ & J^z\hat S^z & \hat I \end{bmatrix} \end{align}

With the special first and last matrices:

\begin{align} \hat W^{\left[1\right]} &= \begin{bmatrix} -h_z\hat S^z-h_x\hat S^x & (J/2)\hat S^- & (J/2)\hat S^+ & J^z\hat S^z & \hat I \end{bmatrix}\\ \hat W^{\left[L\right]} &=\begin{bmatrix} \hat I\newline \hat S^+ \newline \hat S^- \newline \hat S^z\newline -h_z\hat S^z-h_x\hat S^x \end{bmatrix} \end{align}

The $W^{\sigma_i,\sigma_i^\prime}$ can be directly constructed from the physical operators:

\begin{align} \hat W^{\sigma_i\sigma_i^{\prime}}_{j, k} = \langle\sigma_i\lvert\hat W^{\left[i\right]}_{j, k} \lvert\sigma_i'\rangle \end{align}

©: This site was created by Piet Dargel and Thomas Köhler